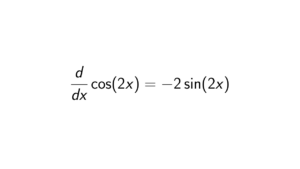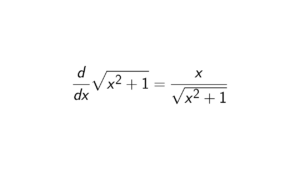Limit of (1+x)^(1/x) as x approaches 0

We want to prove that the limit of

(1+x)^{\frac{1}{x}}

x

approaches 0 is

e

.

Proof. Define

\begin{align*} y = \lim_{x \rightarrow 0} (1 + x)^{\frac{1}{x}} \end{align*}

Take the natural logarithm on both sides. Then we get

\begin{align*} \ln(y) &= \ln\bigg(\lim_{x \rightarrow 0} (1 + x)^{\frac{1}{x}}\bigg) \\ &= \lim_{x \rightarrow 0} \ln\bigg[(1 + x)^{\frac{1}{x}}\bigg] \\ &= \lim_{x \rightarrow 0} \frac{\ln(1 + x)}{x}, \end{align*}

where the last equality follows by the fact that

\ln(A^B) = B\ln(A)

. Now we do see that we get

\begin{align*} \lim_{x \rightarrow 0} \frac{\ln(1 + x)}{x} = \frac{0}{0}. \end{align*}

So we can apply L’ Hopital’s rule:

\begin{align*} \ln(y) &= \lim_{x \rightarrow 0} \frac{\frac{d}{dx}\ln(1 + x)}{\frac{d}{dx} x} \\ &= \lim_{x \rightarrow 0} \frac{\frac{1}{1+x}}{1} \\ &= \lim_{x \rightarrow 0} \frac{1}{1+x} \\ &= \frac{1}{1 + 0} \\ &= 1. \end{align*}

So we get

\begin{align*} \ln(y) = 1 \iff e^{\ln(y)} = e^1 \iff y = e. \end{align*}

Therefore, in conclusion, we get

\begin{align*} y = \lim_{x \rightarrow 0} (1 + x)^{\frac{1}{x}} = e. \end{align*}