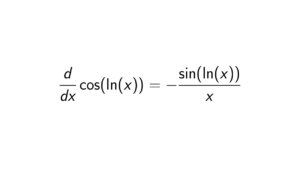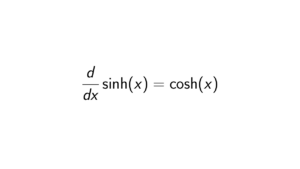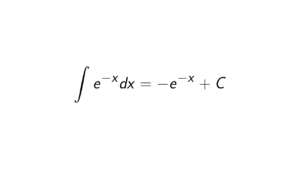For G/N, (gN)^a = g^aN for all integers a

Let $G$ be a group and $N$ be a normal group of $G$ . Then $(gN)^a$ = $g^aN$ for all $a \in \mathbb{Z}$ .

Proof. We know that

uN\cdot vN = (uv)N

for all

u,v \in G

since

N

is a normal subgroup of

G

. This implies that

gN \cdot gN = ggN = g^2N

. If we apply this

a

, then we get

\begin{align*} (gN)^a &= gN \cdot gN \cdot gN \cdots gN \\ &= g^2N \cdot gN \cdots gN \\ & \ \ \vdots \\ &= g^aN, \end{align*}

which proves the statement.