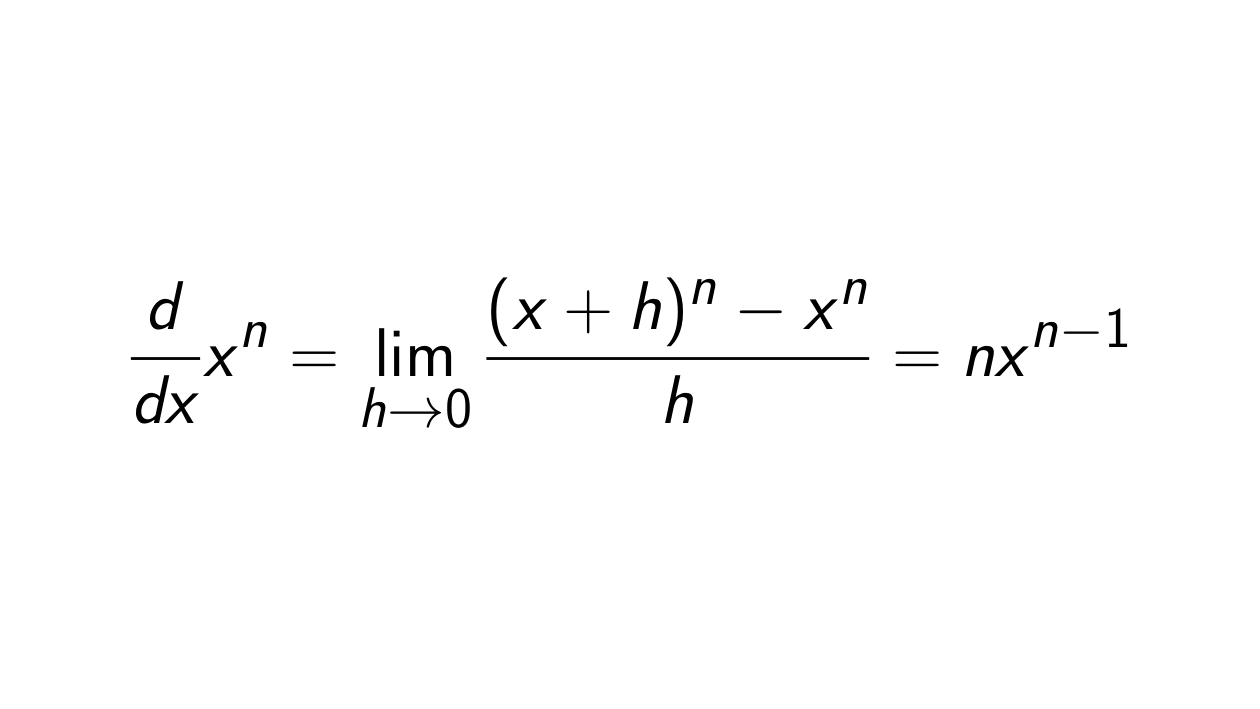Proof. Let f(x) = x^n. Then using the first principle of derivatives, we get:
\begin{align*}
f'(x) &= \lim_{h \rightarrow 0} \frac{f(x+h) - f(x)}{h} \\
&= \lim_{h \rightarrow 0} \frac{(x + h)^n - x^n}{h}.
\end{align*}\begin{align*}
a^n - b^n = (a-b)(a^{n-1} + a^{n-2}b + a^{n-3}b^2 + \cdots + ab^{n-2} + b^{n-1}),
\end{align*}\begin{align*}
f'(x) &= \lim_{h \rightarrow 0} \frac{(x + h)^n - x^n}{h} \\
&= \lim_{h \rightarrow 0} \frac{h[(x+h)^{n-1} + (x+h)^{n-2}x + \cdots + (x+h)x^{n-2} + x^{n-1}]}{h} \\
&= \lim_{h \rightarrow 0} ((x+h)^{n-1} + (x+h)^{n-2}x + \cdots + (x+h)x^{n-2} + x^{n-1}) \\
&= (x+0)^{n-1} + (x+0)^{n-2}x + \cdots + (x+0)x^{n-2} + x^{n-1} \\
&= x^{n-1} + x^{n-2}x + \cdots + xx^{n-2} + x^{n-1} \\
&= x^{n-1} + x^{n-1} + \cdots + x^{n-1} + x^{n-1} \\
&= nx^{n-1}.
\end{align*}