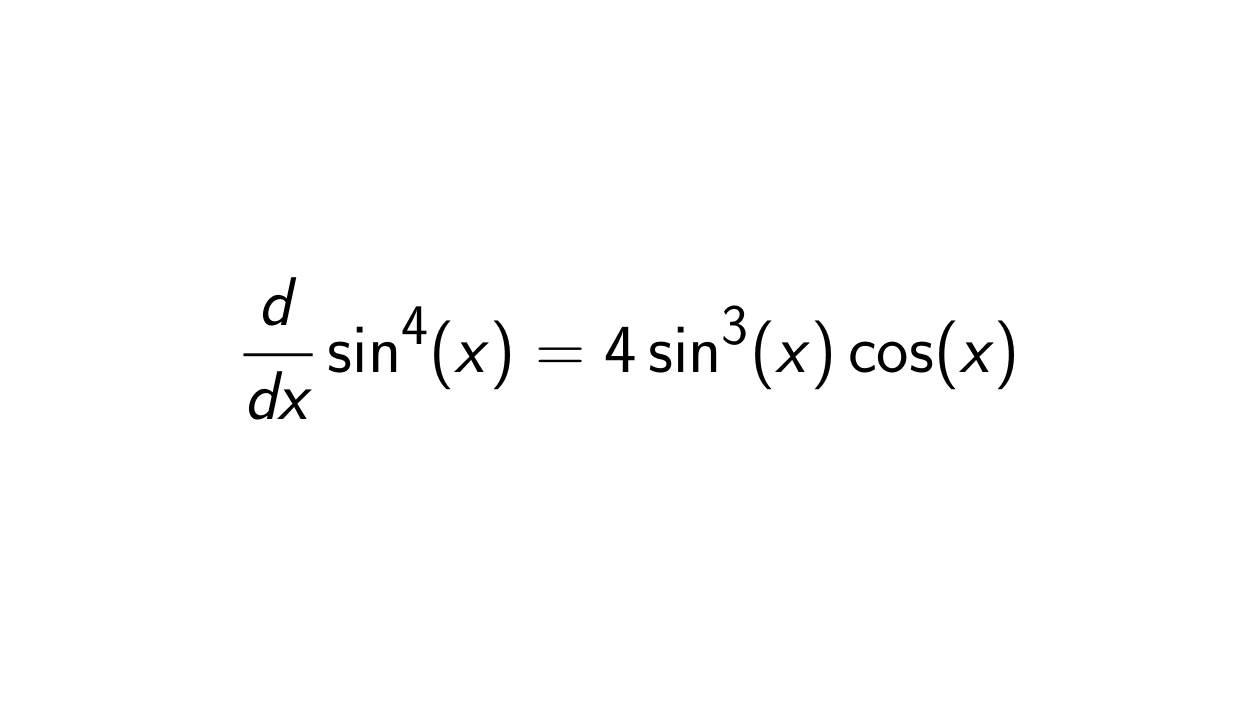What is the derivative of sin^4(x)?

The derivative of \sin^4(x) is 4\sin^3(x)\cos(x).

Solution of the derivative of sin^4(x)

Solution: let F(x) = g(f(x)) = \sin^4(x) where g(u) = u^4 and f(x) = \sin(x). To find the derivative of \sin^4(x), we need to apply the chain rule on F(x):

\begin{equation*}
F'(x) = g'(f(x))f'(x)
\end{equation*}

The derivative of u^4 is 4u^3 and the derivative of \sin(x) is \cos(x), which we have seen here earlier. Therefore, we get:

\begin{equation*}
g'(f(x)) = g'(\sin(x)) = 4\sin^3(x) \text{ and } f'(x) = \cos(x).
\end{equation*}

Substituting everything, we get:

\begin{align*}
F'(x) &= g'(f(x))f'(x) \\
&= 4\sin^3(x)\cos(x)
\end{align*}

Therefore, the derivative of \sin^4(x) is 4\sin^3(x)\cos(x).